
Did you know that it's possible to create intricate works of art using mathematics and computer programs? Many of us use technology in our math classes daily, especially when graphing functions on calculators like the TI series. While these tools are primarily designed for mathematical analysis, they sometimes produce graphs that resemble recognizable shapes, sparking curiosity and creativity in mathematical visualization.

Over time, students and enthusiasts have explored ways to push the boundaries of graphing technology. Some have memorized specific equations or followed templates to recreate familiar shapes, such as hearts, stars, or even simple portraits, on their graphing devices. Others have taken this a step further by layering multiple functions and constraints to create more detailed visual representations. This practice has led to a fascinating intersection between mathematics, programming, and artistic expression.
But beyond simple 2D vector drawings, is it truly possible to generate complex and detailed images using mathematical functions alone? With advancements in graphing software and mathematical modeling, artists and mathematicians alike have experimented with parametric equations, polar coordinates, and fractal geometry to create stunningly intricate designs. Techniques such as Fourier series approximations, Bézier curves, and even AI-driven mathematical art have further expanded the possibilities.
Art By Maths
Zeynep Tezcan
As we continue to explore the relationship between numbers and visuals, it becomes clear that mathematics is not just a tool for problem-solving but also a medium for artistic expression. Could the future of digital art be driven by equations and algorithms?
Recently, a research student from University College London (UCL) successfully created intricate digital art using mathematical equations, along with various programming and graphic techniques. This achievement highlights the fascinating intersection of mathematics, computer science, and visual creativity.
Every digital image we encounter on the internet consists of countless pixels, each assigned a specific color.
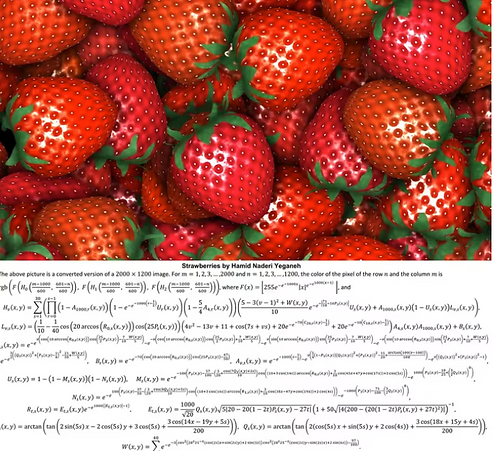
These colors are encoded as numerical data in a digital format, allowing computers to render complex visuals. Beyond conventional computer science knowledge, researchers have explored mathematical concepts like fractals to generate highly detailed and visually stunning images.
A fractal is a mathematical construct that exhibits self-similarity, meaning its intricate patterns repeat at different scales. According to Wikipedia, “In mathematics, a fractal is a geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension.” Fractals can be found in nature, such as in snowflakes, coastlines, and tree branches, but they can also be generated computationally to produce mesmerizing artistic designs.
By leveraging fractal mathematics, researchers have developed algorithms capable of creating infinitely complex structures, revealing how mathematical precision can be transformed into breathtaking digital art. This innovation raises intriguing questions about the future of algorithmic creativity and whether math could become a primary tool for artistic expression in the digital world.
The fusion of mathematics, computer science, and digital art showcases the limitless potential of algorithmic creativity. By harnessing mathematical principles such as fractals, researchers and artists alike can generate intricate, self-replicating patterns that push the boundaries of traditional artistic expression. As technology continues to evolve, the line between science and art becomes increasingly blurred, opening new avenues for innovation in both fields.
